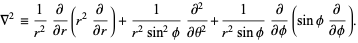 |
(1) |
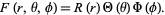 |
(2) |
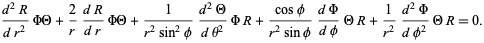 |
(3) |
Now divide by 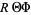 ,
, 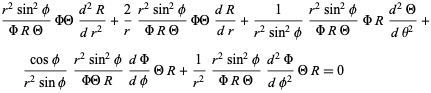 |
(4) |
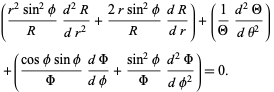 |
(5) |
The solution to the second part of (5) must be sinusoidal, so the differential equation is 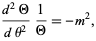 |
(6) |
which has solutions which may be defined either as a complex function with  , ...,
, ..., 
 |
(7) |
or as a sum of real sine and cosine functions with  , ...,
, ..., 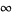
 |
(8) |
Plugging (6) back into (7), 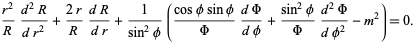 |
(9) |
The radial part must be equal to a constant
 |
(10) |
 |
(11) |
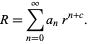 |
(12) |
Then
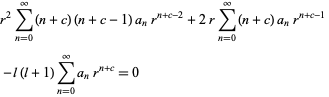 |
(13) |
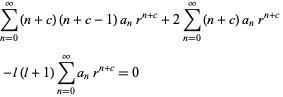 |
(14) |
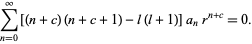 |
(15) |
This must hold true for all powers of 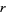 . For the
. For the 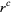 term (with
term (with 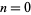 ),
), 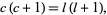 |
(16) |
which is true only if  and all other terms vanish. So
and all other terms vanish. So 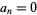 for
for 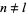 ,
, 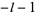 . Therefore, the solution of the
. Therefore, the solution of the 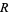 component is given by
component is given by 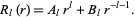 |
(17) |
Plugging (17) back into (◇), 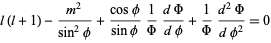 |
(18) |
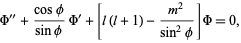 |
(19) |
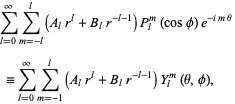 |
(20) |
where
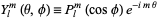 |
(21) |
 |
(22) |
Some of the normalization constants of 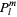 can be absorbed by
can be absorbed by  and
and 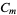 , so this equation may appear in the form
, so this equation may appear in the form  |
(23) |
where
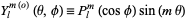 |
(24) |
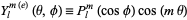 |
(25) |
انا صراحة ماعرف شو لابلاس لاكن شفتلك من الانترنيت
,
,
, and the separation functions are
,
,
, giving a Stäckel determinant of
.
,
, ...,
, ...,
. For the
term (with
),
and all other terms vanish. So
for
,
. Therefore, the solution of the
component is given by
and
, ...,
. The general complex solution is therefore
can be absorbed by
and
, so this equation may appear in the form
is constant and the solution of the
component is a Legendre polynomial
. The general solution is then







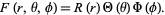
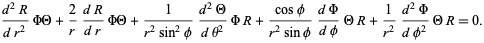
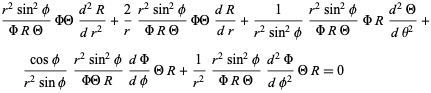
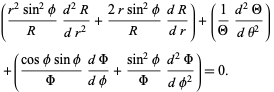
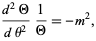


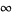

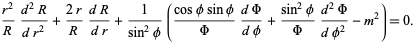


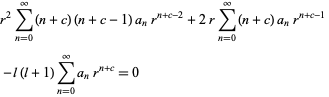
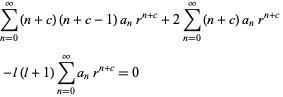
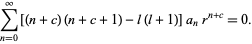
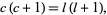
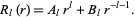
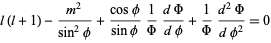
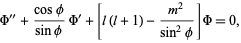
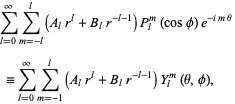
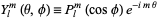


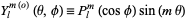
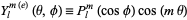
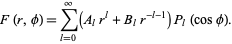

 رد مع اقتباس
رد مع اقتباس
مواقع النشر (المفضلة)